Squaring the Circle – A new method using the Vesica Pisces
Frank has discovered a new way to square the circle so that the perimeter of the square and the circumference of the circle are almost exactly equal. His method uses only a straight-edge and compass, and is the first to work from the inside out using the Vesica Pisces. The protocol is as follows (see the animated image below for visual reference):
1: Draw a circle (#1).
2: Open your compass to the diameter of circle #1, and draw a second circle (#2) (that has a radius exactly twice the original circle), whose center lies on the perimeter of circle #1.
3: Draw a light line (m) connecting the center of circles #1 and #2, projecting it across most of the page.
4: Use where this line m meets the opposite side of circle #1 to be the center of a new circle, with the same radius as circle #2, this is circle #3.
5: Circles #2 and #3 form the Vesica Pisces, and intersect each other in two new points. Connect these points with a line (n), which is orthogonal to line m.
6: Where line n intersects circle #1 it creates two new points. Use these as the centers of two new circles with the same radius as Circles #2 and #3; let point Q be the center of circle #4 and point R be the center of circle #5. These new circles, #4 and #5, form another Vescia Pisces at 90 degrees to the first. The two Vescia Pisces cradle circle #1 where they overlap.
7: Circles #4 and #5 intersect in two points that lie on line m. Choose one of these points (P), and draw a line (s) orthogonal (at 90 degrees) to line m (and thus parallel to line n) at this point. From point P, extend line s on either side of line m so that its total length is just larger than the diameter of circle #1.
8: Note the center points of circles #4 and #5, Q and R. From each of these center ponts draw a line perpendicular to line n (parallel to line m), extending them to meet line s in two new points (S and T). Let S be the point made by extending from point Q and T be the point made from extending point R.
9: Open your compass to the length made between points S and R, and draw a circle (#6) of that radius with its center on point R.
10: Draw another circle (#7) of the same radius, with its center on point Q.
11: Circles #6 and #7 intersect line n in two new points (U and V) towards the outsides of the construction. Open your compass to the length made between the center of circle #1 and one of these new points, and draw a new circle (#8) with the same center as circle #1.
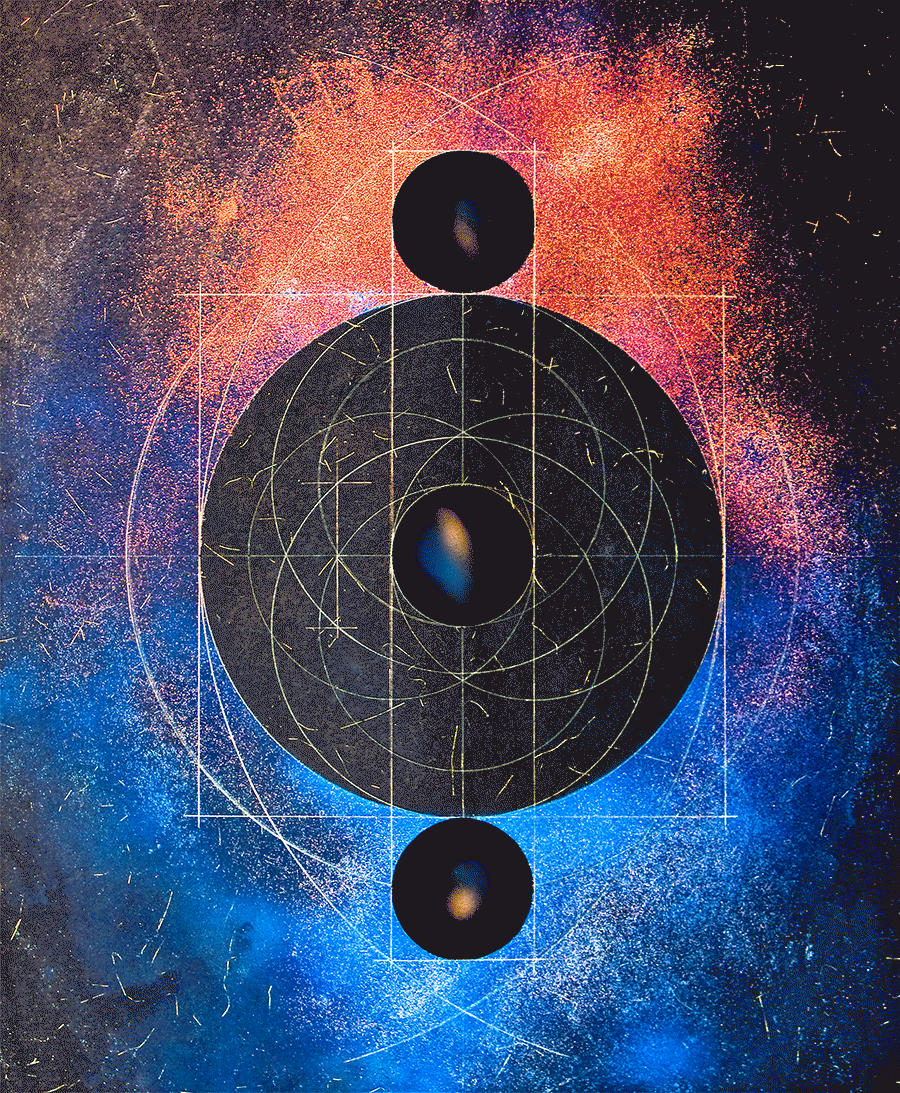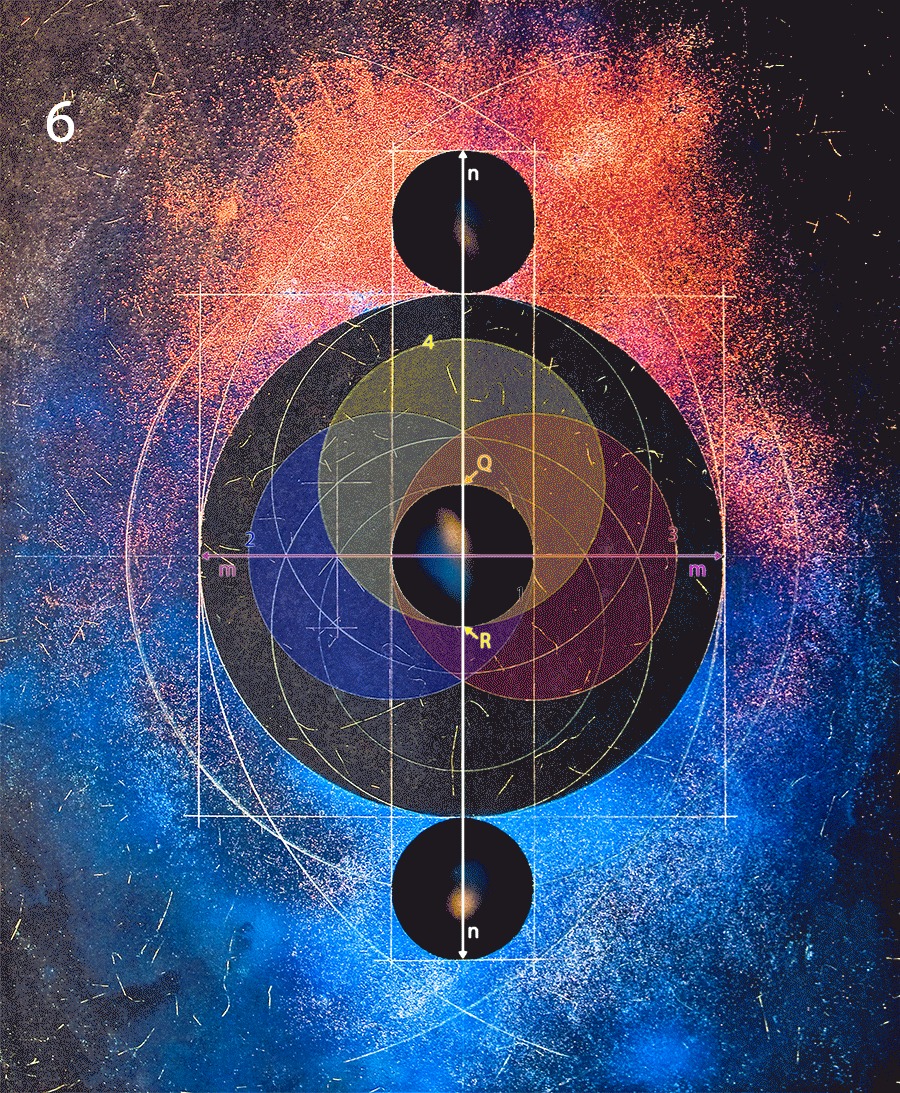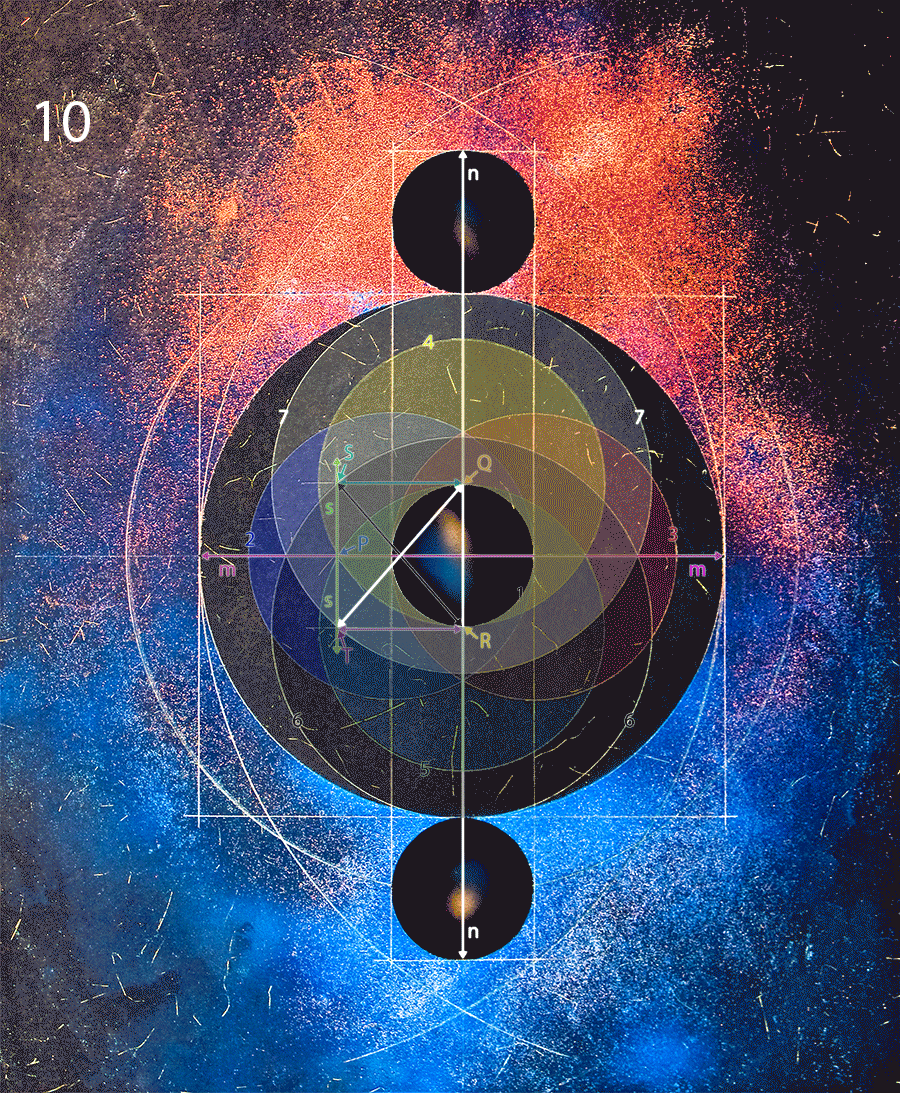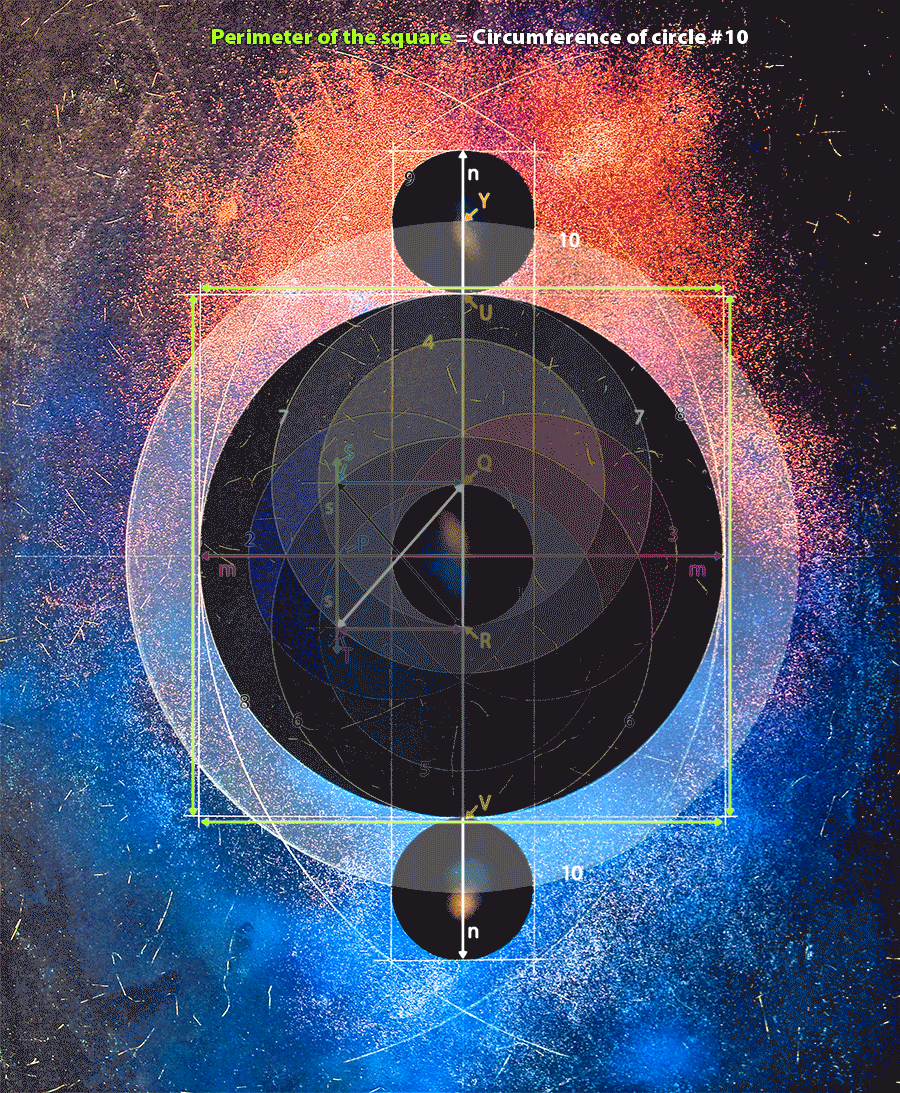
12: Circle #8 meets lines m and n in four points. Construct a total four lines (one on each point), two parallel to n and two parallel to m, such that the lines form a square in which circle #8 is circumscribed.
13: Open your compass to the original radius of circle #1. With the point of your compass on point U, mark a new point (Y) on line n towards the outside of the construction.
14: Draw a new circle (#9) with a center on point Y, with a radius equal to circle #1. Circle #9 is tangent to circle #8.
15: Open your compass to the distance between the centers of circles #1 and #9, and draw a final circle (#10) with the same center as circle #1. This circle has a perimeter almost exactly equal to the square constructed in step 12.
The mathematics of this construction are known exactly. Measurements of the circumference of the circle and the perimeter of the square agree to within 99.9+%, with the perimeter of the square being ever so slightly smaller than the circumference of the circle. It was proven in 1882 that it is impossible to perfectly square the circle using a finite number of steps with a straightedge and compass. This is a result of the Lindemann-Weierstrass theorem, which was involved in showing that the number pi is transcendental, and thus a non-algebraic number. Straightedge and compass constructions involving non-algebraic numbers can only be approximations.
You must be logged in to post a comment.